Unveiling The World From A Single Point: A Comprehensive Guide To Azimuthal Equidistant Projections

Unveiling the World from a Single Point: A Comprehensive Guide to Azimuthal Equidistant Projections

Related Articles: Unveiling the World from a Single Point: A Comprehensive Guide to Azimuthal Equidistant Projections

Introduction

With great pleasure, we will explore the intriguing topic related to Unveiling the World from a Single Point: A Comprehensive Guide to Azimuthal Equidistant Projections. Let’s weave interesting information and offer fresh perspectives to the readers.

Unveiling the World from a Single Point: A Comprehensive Guide to Azimuthal Equidistant Projections

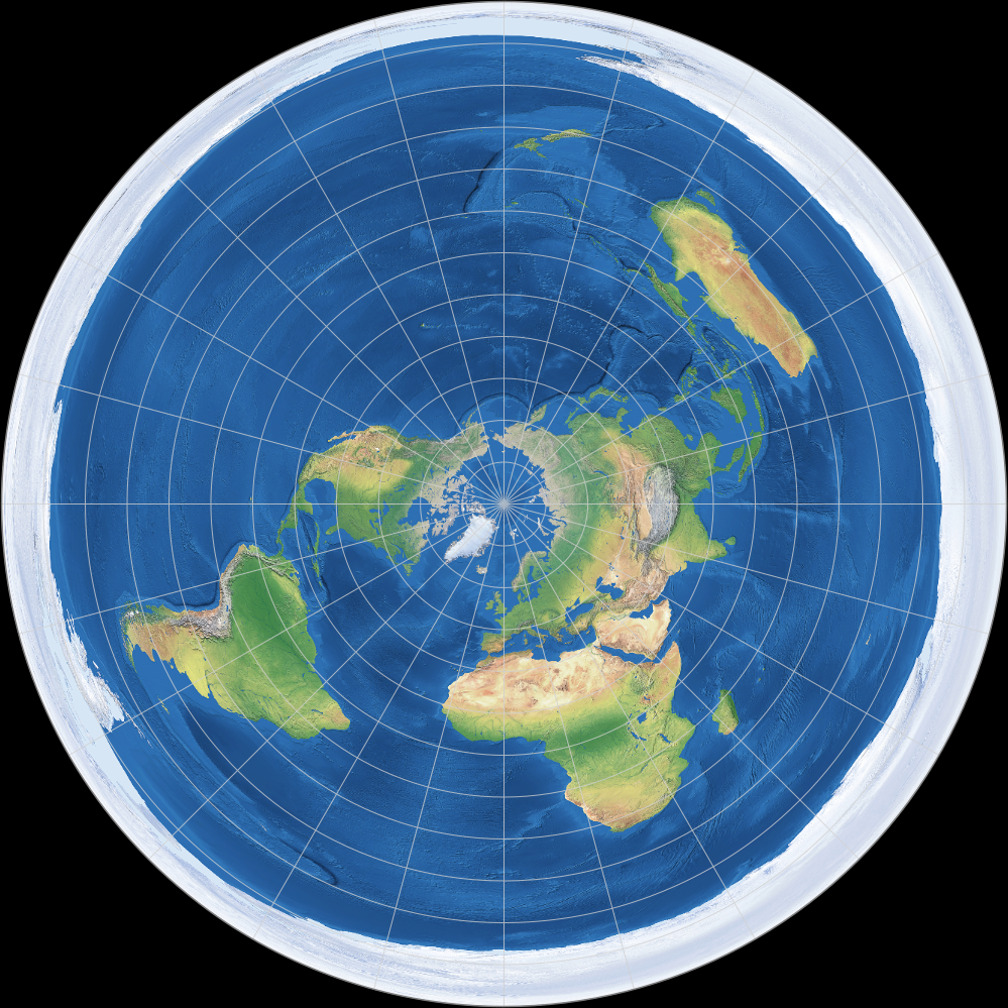
The Earth, a sphere suspended in space, presents a challenge for cartographers seeking to represent its complex curvature on a flat surface. Various map projections have emerged to address this challenge, each with its own strengths and limitations. Among these, the azimuthal equidistant projection stands out for its unique ability to accurately depict distances from a chosen central point while preserving true directions (azimuths) from that point. This projection, though not without its drawbacks, finds applications in a diverse range of fields, from navigation and military operations to geographical education and data visualization.

Understanding the Fundamentals:

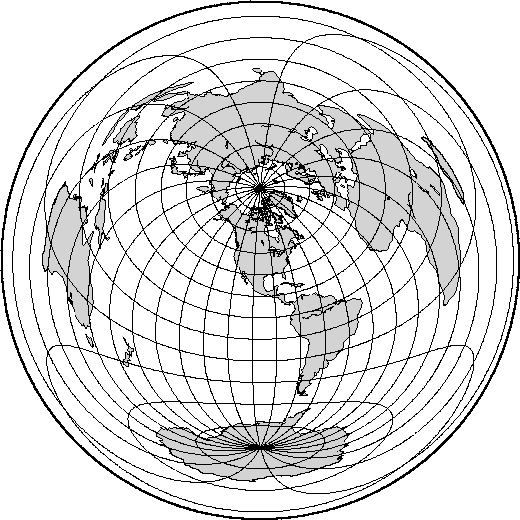
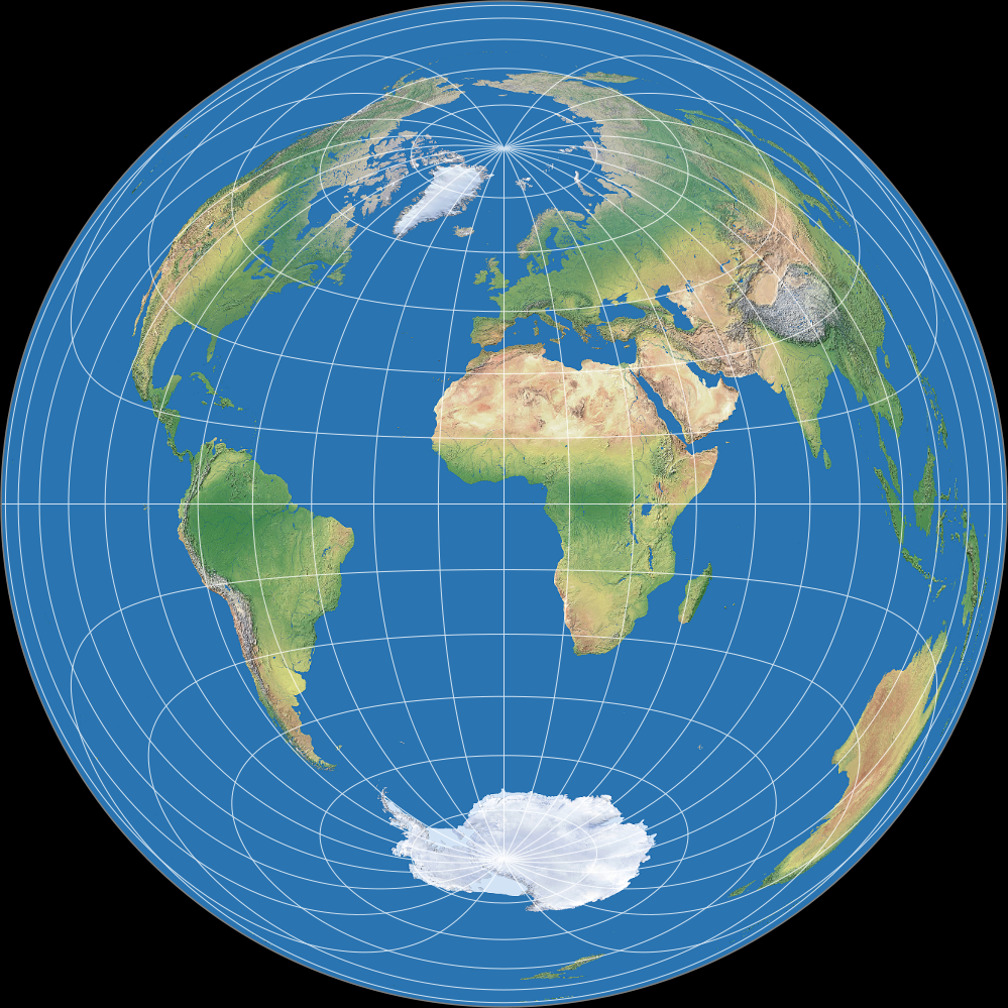
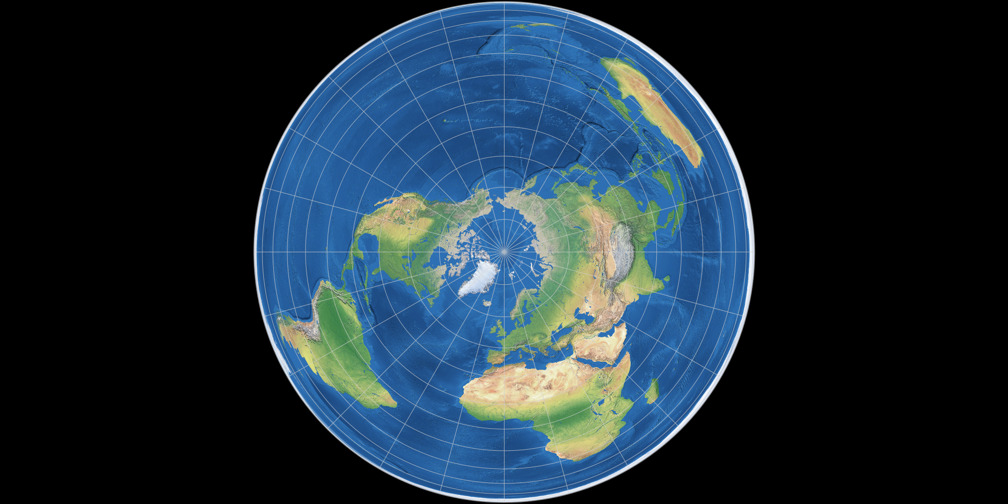
The azimuthal equidistant projection, as its name suggests, is a type of map projection that preserves both azimuths and distances from a central point. This central point, known as the "projection point," can be any location on the Earth’s surface. The projection is constructed by imagining a sphere representing the Earth, with the projection point situated at the center. Lines of longitude and latitude are then projected onto a flat plane tangent to the sphere at the projection point.

Key Features and Characteristics:

The azimuthal equidistant projection exhibits several key features that distinguish it from other map projections:

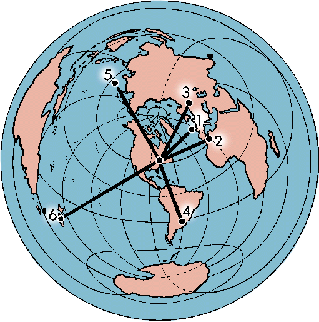
• True Azimuths: The projection accurately preserves directions from the central point. This means that lines drawn from the central point to any other location on the map represent the true compass bearing between those two points.
• Accurate Distances: Distances measured from the central point to any other location on the map are represented at their true scale. This property makes the projection suitable for applications where precise distance measurements are crucial.
• Distortion: While distances and directions are preserved from the central point, the projection introduces significant distortion in areas farther away from the projection point. Shapes and sizes of landmasses are increasingly distorted as they move further from the central point.
• Types of Azimuthal Equidistant Projections: There are three primary types of azimuthal equidistant projections:
  • Planar: The projection point is located at the North or South Pole, resulting in a circular map where distances are measured from the pole.
  • Polar: The projection point is located on the equator, resulting in a circular map where distances are measured from the chosen point on the equator.
  • Oblique: The projection point is located anywhere on the Earth’s surface, resulting in a circular map where distances are measured from that specific point.

Applications and Uses:

The azimuthal equidistant projection, despite its inherent distortions, finds applications in various fields due to its unique properties:

• Navigation: The projection’s ability to accurately represent distances and directions from a central point makes it useful for navigation, particularly for air and sea travel. It allows pilots and sailors to accurately calculate distances and bearings for their routes.
• Military Operations: Military planners utilize the projection for strategic planning and operations, as it allows them to accurately assess distances and directions from a specific location.
• Geographical Education: The projection is frequently used in educational settings to illustrate the concept of distance and direction from a particular point on the Earth’s surface. It helps students visualize how distances and directions change as they move away from a central point.
• Data Visualization: The projection can be employed to visually represent data that is associated with a particular location. For example, it can be used to visualize the distribution of population, temperature, or rainfall around a specific city or region.

Limitations and Considerations:

While the azimuthal equidistant projection offers valuable advantages, it also has limitations that must be considered:

• Distortion: The projection introduces significant distortion in areas far away from the central point. Shapes and sizes of landmasses are distorted, making it unsuitable for representing the entire Earth accurately.
• Limited Use for Global Representation: The projection is primarily useful for representing a specific area or region centered around the projection point. It is not suitable for representing the entire globe accurately.
• Not Suitable for Area Comparisons: The projection distorts areas, making it unsuitable for comparing the relative sizes of different regions.

Frequently Asked Questions (FAQs):

Q: What is the difference between an azimuthal equidistant projection and a Mercator projection?

A: The Mercator projection preserves angles and shapes but distorts areas and distances, particularly towards the poles. The azimuthal equidistant projection preserves distances and directions from a central point but distorts shapes and areas away from that point.

Q: What is the best way to use an azimuthal equidistant projection?

A: The projection is best suited for representing a specific area or region centered around the projection point. It is useful for navigation, military operations, and data visualization related to a specific location.

Q: What are the limitations of using an azimuthal equidistant projection?

A: The projection introduces significant distortion in areas far away from the central point, making it unsuitable for representing the entire globe accurately or for comparing the relative sizes of different regions.

Tips for Using Azimuthal Equidistant Projections:

• Choose the Appropriate Projection Point: Select a projection point that aligns with the specific area or region of interest.
• Consider the Scale: The projection’s accuracy in representing distances and directions depends on the scale of the map.
• Be Aware of Distortion: Understand the limitations of the projection and be aware of the distortion introduced in areas farther away from the central point.
• Use in Conjunction with Other Projections: The azimuthal equidistant projection can be used in conjunction with other projections to create composite maps that combine the advantages of different projections.

Conclusion:

The azimuthal equidistant projection, while not a perfect representation of the Earth’s surface, offers a unique perspective on the world by preserving distances and directions from a central point. Its applications extend across diverse fields, from navigation and military operations to geographical education and data visualization. Understanding its strengths and limitations allows for its effective use in various contexts, providing valuable insights into the complexities of mapping our planet.

Closure

Thus, we hope this article has provided valuable insights into Unveiling the World from a Single Point: A Comprehensive Guide to Azimuthal Equidistant Projections. We appreciate your attention to our article. See you in our next article!