Unveiling The World: A Comprehensive Guide To Three Major Map Projections

Unveiling the World: A Comprehensive Guide to Three Major Map Projections

Related Articles: Unveiling the World: A Comprehensive Guide to Three Major Map Projections

Introduction

With great pleasure, we will explore the intriguing topic related to Unveiling the World: A Comprehensive Guide to Three Major Map Projections. Let’s weave interesting information and offer fresh perspectives to the readers.

Unveiling the World: A Comprehensive Guide to Three Major Map Projections

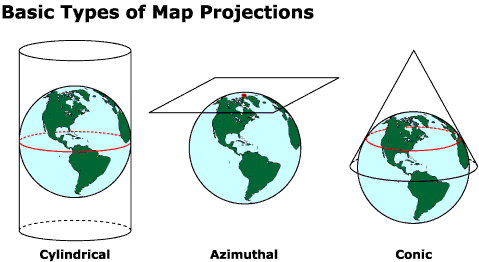
The Earth, a sphere suspended in space, presents a unique challenge to cartographers: how to accurately represent its three-dimensional surface on a two-dimensional plane. This challenge has led to the development of numerous map projections, each employing distinct mathematical formulas to transform the Earth’s curvature onto a flat surface. While no single projection can perfectly capture all aspects of the globe, certain projections excel in specific applications. This exploration delves into the intricacies of three major map projections: Mercator, Robinson, and Winkel Tripel, highlighting their strengths, weaknesses, and the contexts in which they are most effectively employed.

1. The Mercator Projection: A Legacy of Navigation

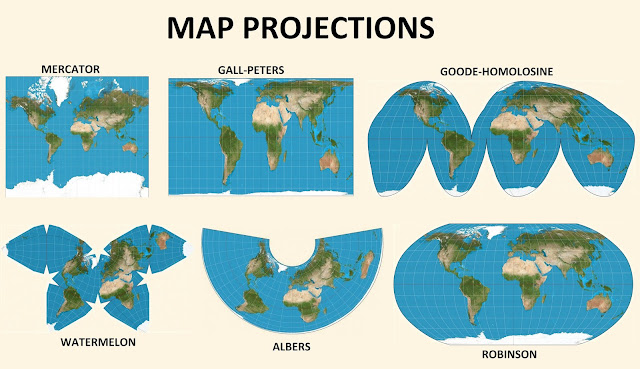
The Mercator projection, developed by Flemish cartographer Gerardus Mercator in 1569, is perhaps the most recognizable map projection. Its defining characteristic lies in its preservation of angles, making it a valuable tool for navigation. This property ensures that compass bearings remain accurate, a crucial feature for sailors charting their courses across the vast oceans.

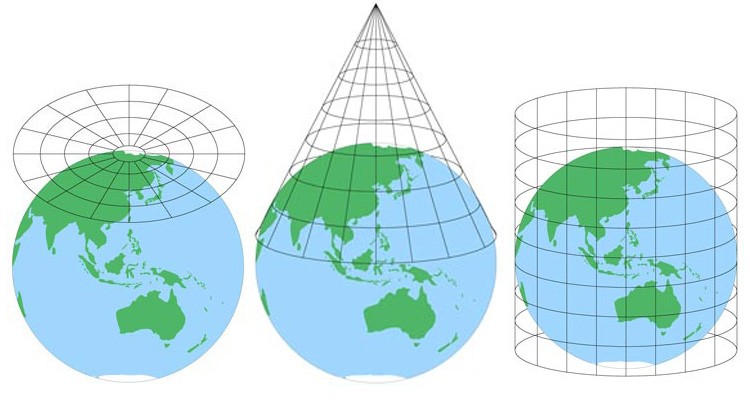
How it Works: The Mercator projection employs a cylindrical projection, imagining the Earth enveloped in a cylinder tangent to the equator. Lines of longitude are projected as vertical lines, while lines of latitude are projected as horizontal lines, increasing in spacing as they move away from the equator. This systematic distortion, a consequence of the transformation from a sphere to a cylinder, leads to a significant exaggeration of landmasses at higher latitudes. Greenland, for instance, appears larger than South America on a Mercator projection, despite being considerably smaller in reality.

Benefits:

• Preserves angles: Ideal for navigation as compass bearings are accurately represented.
• Rectangular grid: Facilitates the use of coordinates for precise location identification.
• Familiarity: Widespread use has made it the most recognizable map projection.

Drawbacks:

• Distorted area: Regions at higher latitudes are significantly exaggerated, leading to a misrepresentation of relative size.
• Distorted shape: Landmasses near the poles appear elongated and distorted.

Applications:

• Navigation: Used extensively in maritime and aviation charts due to its angle preservation.
• Atlases and textbooks: Frequently employed for general world maps, albeit with caveats regarding area distortion.
• Geographic Information Systems (GIS): Used in some applications, though not as common as other projections.

2. The Robinson Projection: A Balanced Approach

Introduced in 1963 by Arthur H. Robinson, the Robinson projection seeks to minimize distortions in both area and shape, offering a more balanced representation of the Earth. It achieves this by employing a compromise between cylindrical and conic projections, incorporating a unique mathematical formula to project the globe onto an oval-shaped surface.

How it Works: The Robinson projection, unlike the Mercator, doesn’t maintain true angles. Instead, it aims to strike a balance by introducing controlled distortions to minimize overall error. Lines of longitude converge at the poles, while lines of latitude are curved, becoming more compressed towards the poles. This approach results in a map that is visually appealing and generally less distorted than the Mercator projection.

Benefits:

• Reduced area distortion: Landmasses are represented with greater accuracy in terms of relative size compared to the Mercator projection.
• Improved shape representation: Landmasses are less distorted, particularly near the poles.
• Visually appealing: The oval shape and balanced distortions create a more aesthetically pleasing map.

Drawbacks:

• Compromised angles: Angles are not preserved, making it less suitable for navigation.
• Not a true projection: The Robinson projection is not a true mathematical projection but rather an approximation, leading to some inaccuracies.

Applications:

• General purpose maps: Frequently used in atlases, textbooks, and media for its balanced representation of the globe.
• Educational materials: Offers a more accurate depiction of the Earth compared to the Mercator projection, making it suitable for educational purposes.
• Global overview maps: Provides a visually appealing and relatively accurate representation for global overviews.

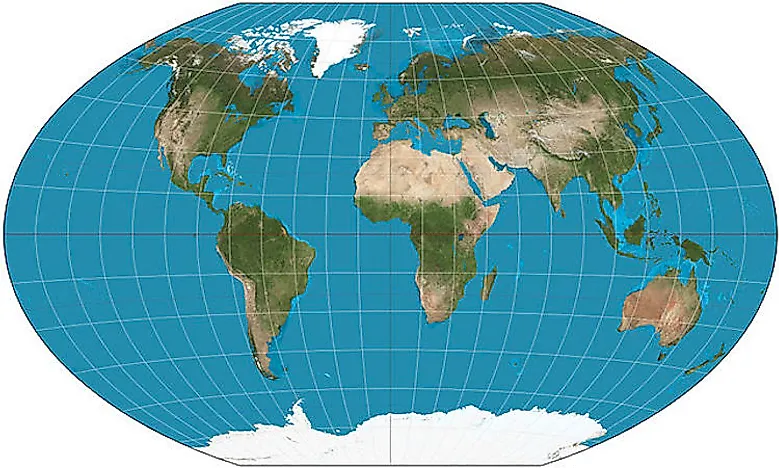
3. The Winkel Tripel Projection: A Quest for Accuracy

The Winkel Tripel projection, developed by Oswald Winkel in 1921, is a compromise projection designed to minimize distortions in both area and shape, particularly in the polar regions. It combines elements of the Mercator and Aitoff projections, resulting in a map that is both visually appealing and relatively accurate.

How it Works: The Winkel Tripel projection uses a combination of equidistant and azimuthal projections to project the globe onto a modified conic surface. Lines of longitude are equally spaced, while lines of latitude are curved, becoming more compressed towards the poles. This approach effectively reduces area distortion, particularly in the polar regions, while also minimizing shape distortion.

Benefits:

• Minimized area distortion: Landmasses are represented with greater accuracy in terms of relative size, especially in the polar regions.
• Reduced shape distortion: Landmasses are less distorted compared to the Mercator projection, particularly at higher latitudes.
• Visually appealing: The balanced distortions create a pleasing and relatively accurate representation of the Earth.

Drawbacks:

• Compromised angles: Angles are not preserved, making it less suitable for navigation.
• Complex mathematical formula: The Winkel Tripel projection involves complex mathematical calculations, making it more computationally intensive than simpler projections.

Applications:

• World maps: Frequently used in atlases, textbooks, and media for its balanced representation of the Earth.
• Educational materials: Offers a more accurate depiction of the Earth compared to the Mercator projection, making it suitable for educational purposes.
• Global overview maps: Provides a visually appealing and relatively accurate representation for global overviews.

FAQs: A Deeper Dive into the Projections

Mercator Projection:

• Q: Why is Greenland so large on a Mercator projection?
  • A: The Mercator projection significantly exaggerates areas at higher latitudes due to its cylindrical nature. This distortion makes Greenland appear larger than South America, despite being considerably smaller in reality.
• Q: Is the Mercator projection still relevant in the age of GPS?
  • A: While GPS provides precise location information, the Mercator projection remains relevant for maritime and aviation navigation due to its angle preservation, which ensures accurate compass bearings.
• Q: What are the alternatives to the Mercator projection?
  • A: The Robinson, Winkel Tripel, and other projections offer less distorted representations of the Earth, though they may not be as suitable for navigation.

Robinson Projection:

• Q: What makes the Robinson projection a "compromise" projection?
  • A: The Robinson projection aims to balance area and shape distortions by employing a unique mathematical formula that incorporates elements of both cylindrical and conic projections.
• Q: Why is the Robinson projection not considered a "true" projection?
  • A: The Robinson projection involves approximations in its mathematical formula, leading to some inaccuracies in its representation of the Earth.
• Q: What are the limitations of the Robinson projection?
  • A: The Robinson projection does not preserve angles, making it less suitable for navigation. It also involves some distortions, though these are generally minimized compared to the Mercator projection.

Winkel Tripel Projection:

• Q: What makes the Winkel Tripel projection particularly suitable for world maps?
  • A: The Winkel Tripel projection offers a balanced representation of the Earth with minimal area and shape distortions, making it ideal for world maps and global overviews.
• Q: How does the Winkel Tripel projection minimize distortions in the polar regions?
  • A: The Winkel Tripel projection combines elements of the Mercator and Aitoff projections, effectively reducing area distortion in the polar regions while also minimizing shape distortion.
• Q: What are the trade-offs associated with the Winkel Tripel projection?
  • A: The Winkel Tripel projection, while minimizing distortions, does not preserve angles and involves complex mathematical calculations, making it more computationally intensive than simpler projections.

Tips for Using Map Projections:

• Understand the purpose of the map: Consider the specific application of the map before choosing a projection. For navigation, the Mercator projection is ideal, while for general world maps, the Robinson or Winkel Tripel projections offer balanced representations.
• Be aware of distortions: Every map projection introduces distortions. It is crucial to understand the nature of these distortions and their impact on the map’s interpretation.
• Use multiple projections: Combining different projections can provide a more comprehensive understanding of the Earth’s surface. For example, using a Mercator projection for navigation alongside a Robinson projection for general reference can offer a balanced perspective.
• Consult with experts: When working with maps for critical applications, seek guidance from cartographers or GIS professionals to ensure the most appropriate projection is used.

Conclusion: A World of Projections

The three map projections explored – Mercator, Robinson, and Winkel Tripel – highlight the diverse approaches cartographers employ to represent the Earth on a flat surface. Each projection possesses distinct strengths and weaknesses, making it essential to choose the most appropriate projection based on the specific application. While no single projection can perfectly capture the Earth’s spherical form, these projections provide valuable tools for understanding and navigating our planet, revealing a world of possibilities through their unique perspectives.

Closure

Thus, we hope this article has provided valuable insights into Unveiling the World: A Comprehensive Guide to Three Major Map Projections. We thank you for taking the time to read this article. See you in our next article!