Unveiling the World: A Comprehensive Guide to Map Projections
Related Articles: Unveiling the World: A Comprehensive Guide to Map Projections
Introduction
With great pleasure, we will explore the intriguing topic related to Unveiling the World: A Comprehensive Guide to Map Projections. Let’s weave interesting information and offer fresh perspectives to the readers.
Table of Content
Unveiling the World: A Comprehensive Guide to Map Projections
![]()
The Earth, a sphere of unparalleled beauty and complexity, presents a unique challenge for cartographers: how to represent its three-dimensional surface accurately on a two-dimensional map. This is where map projections come into play. A map projection is a systematic transformation of the Earth’s spherical surface onto a flat plane, allowing us to visualize and analyze geographical information. Each projection involves a specific mathematical formula that determines how lines of longitude and latitude are represented, ultimately shaping the map’s appearance and the distortions it introduces.
The Fundamental Challenge of Flattening a Sphere
The Earth’s curvature inherently poses a challenge for accurate representation. Any attempt to flatten a sphere onto a plane inevitably leads to distortions in either shape, area, distance, or direction. This is because the Earth’s surface is curved, while a map is flat. Therefore, map projections are not perfect representations of reality but rather compromises designed to prioritize specific properties depending on the intended use of the map.
Classifying Projections: A Framework for Understanding
Map projections can be classified based on their geometric properties, the shape of their developable surface, and the location of the point of tangency or intersection between the globe and the projection plane. This framework provides a systematic approach for understanding the strengths and limitations of different projections.
1. Geometric Properties:
- Conformal Projections: Preserve angles and shapes locally, meaning small shapes on the map accurately reflect their real-world counterparts. However, conformal projections distort areas, making them unsuitable for representing the true size of continents or countries.
- Equal-Area Projections: Maintain the accurate representation of area, ensuring that the relative sizes of geographical features are preserved. However, equal-area projections distort shapes, making them less suitable for navigation or measuring distances.
- Equidistant Projections: Preserve distances from a specific point, typically the center of the map, to all other points. These projections are useful for measuring distances but introduce distortions in shape and area.
- Compromise Projections: Aim to minimize distortions in multiple properties, offering a balance between shape, area, and distance preservation. These projections are versatile but may not excel in any single property.
2. Developable Surfaces:
- Cylindrical Projections: Imagine wrapping a cylinder around the globe, projecting the Earth’s surface onto the cylinder. This results in maps with straight meridians and parallels that intersect at right angles. Examples include the Mercator projection and the Transverse Mercator projection.
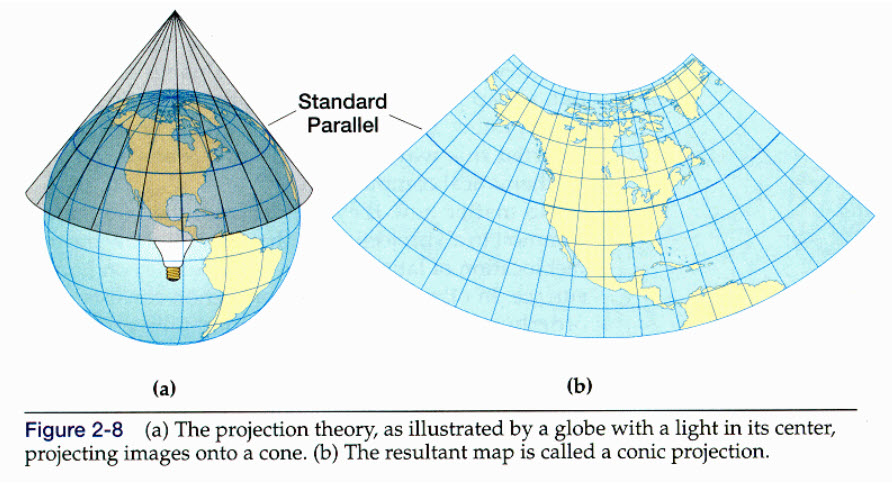
- Conic Projections: Derived from projecting the Earth’s surface onto a cone, conic projections typically have curved meridians and parallel lines that converge at a point. These projections are particularly well-suited for representing mid-latitude regions. Examples include the Albers Equal-Area Conic projection and the Lambert Conformal Conic projection.
- Planar Projections: Also known as azimuthal projections, these projections are created by projecting the Earth’s surface onto a flat plane tangent to the globe at a specific point. They are particularly useful for representing polar regions. Examples include the Stereographic projection and the Gnomonic projection.
3. Point of Tangency or Intersection:
- Tangential Projections: The projection plane touches the globe at a single point, resulting in a map centered on that point.
- Secant Projections: The projection plane intersects the globe along two parallel circles, resulting in maps that represent regions between these circles more accurately.
Popular Map Projections: A Glimpse into the Diverse World of Cartography
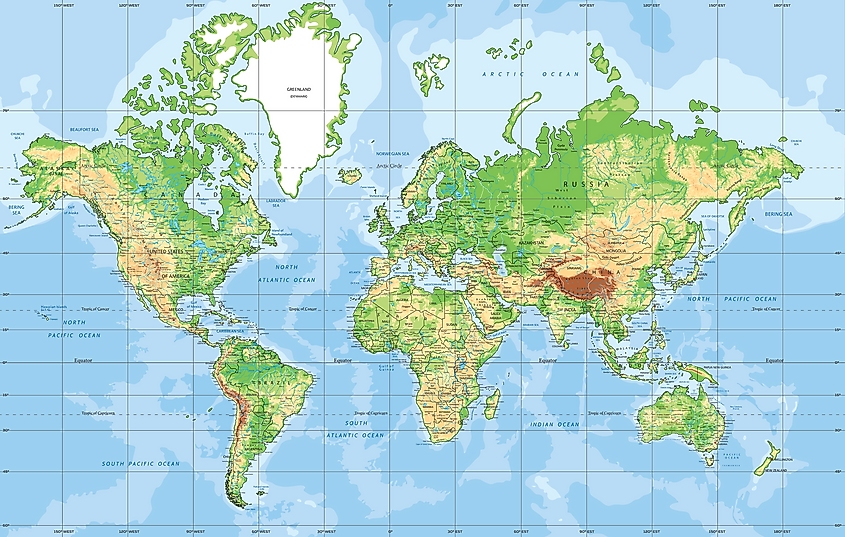
- Mercator Projection: This conformal projection is perhaps the most widely recognized map projection. It is renowned for its preservation of angles and shapes, making it ideal for navigation. However, its significant distortion of areas near the poles, particularly Greenland and Antarctica, has been subject to criticism.
- Robinson Projection: A compromise projection that aims to minimize distortions in shape, area, and distance. The Robinson projection offers a balanced representation of the Earth, making it suitable for general-purpose maps.
- Winkel Tripel Projection: Another compromise projection that aims to balance distortions in shape, area, and distance. The Winkel Tripel projection is commonly used for world maps and atlases due to its relatively low distortions.
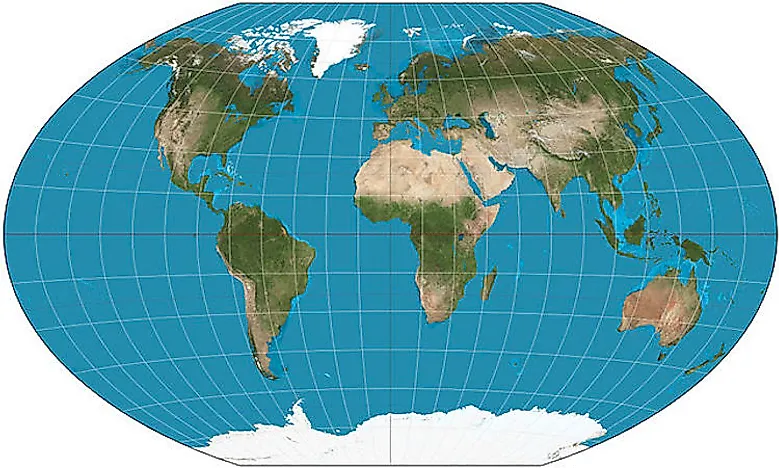
- Mollweide Projection: An equal-area projection that preserves the relative sizes of geographical features. The Mollweide projection is particularly useful for visualizing global patterns and distributions.
- Goode Homolosine Projection: An equal-area projection that uses an interrupted projection technique, breaking up the map into sections to minimize distortions. The Goode Homolosine projection is well-suited for representing continents and their relative sizes accurately.
FAQs by Map Projection Types
1. Mercator Projection
- Q: What are the advantages of the Mercator projection?
- A: Its primary advantage is its preservation of angles and shapes, making it ideal for navigation.
- Q: What are the disadvantages of the Mercator projection?
- A: It significantly distorts areas near the poles, exaggerating the size of Greenland and Antarctica.
- Q: Why is the Mercator projection still widely used?
- A: Its ease of use and familiar appearance make it a popular choice for navigation and mapping applications.
2. Robinson Projection
- Q: What are the advantages of the Robinson projection?
- A: It offers a balanced representation of the Earth with minimal distortions in shape, area, and distance.
- Q: What are the disadvantages of the Robinson projection?
- A: It does not preserve any property perfectly, making it less suitable for specialized applications.
- Q: When is the Robinson projection most suitable?
- A: It is a good choice for general-purpose maps, atlases, and educational materials.
3. Winkel Tripel Projection
- Q: What are the advantages of the Winkel Tripel projection?
- A: It minimizes distortions in shape, area, and distance, offering a balanced representation of the Earth.
- Q: What are the disadvantages of the Winkel Tripel projection?
- A: It does not preserve any property perfectly, making it less suitable for specialized applications.
- Q: When is the Winkel Tripel projection most suitable?
- A: It is commonly used for world maps and atlases due to its balanced distortions.
4. Mollweide Projection
- Q: What are the advantages of the Mollweide projection?
- A: It preserves the relative sizes of geographical features, making it ideal for visualizing global patterns and distributions.
- Q: What are the disadvantages of the Mollweide projection?
- A: It distorts shapes, particularly near the poles, making it less suitable for navigation or measuring distances.
- Q: When is the Mollweide projection most suitable?
- A: It is well-suited for representing global data, such as population density or climate patterns.
5. Goode Homolosine Projection
- Q: What are the advantages of the Goode Homolosine projection?
- A: It preserves the relative sizes of continents and minimizes distortions in shape and area.
- Q: What are the disadvantages of the Goode Homolosine projection?
- A: It uses an interrupted projection technique, breaking up the map into sections, which can be visually disruptive.
- Q: When is the Goode Homolosine projection most suitable?
- A: It is well-suited for representing continents and their relative sizes accurately.
Tips by Map Projection Types
1. Mercator Projection:
- Use it for navigation and applications where preserving angles and shapes is crucial.
- Be aware of its significant area distortions near the poles, particularly for Greenland and Antarctica.
2. Robinson Projection:
- Consider it for general-purpose maps and atlases where a balance of distortions is desired.
- Avoid it for applications requiring precise measurements of area, distance, or shape.
3. Winkel Tripel Projection:
- Use it for world maps and atlases where a balanced representation of the Earth is prioritized.
- Be aware that it does not perfectly preserve any property, making it less suitable for specialized applications.
4. Mollweide Projection:
- Use it for visualizing global patterns and distributions where preserving area is essential.
- Avoid it for navigation or applications requiring accurate measurements of distances or shapes.
5. Goode Homolosine Projection:
- Use it for representing continents and their relative sizes accurately.
- Be aware of its interrupted projection technique, which can be visually disruptive.
Conclusion
Map projections are essential tools for representing the Earth’s surface on flat maps. They offer a diverse range of options, each with its strengths and limitations. Understanding the different types of projections and their inherent distortions is crucial for making informed decisions about which projection best suits a specific purpose. Whether for navigation, data visualization, or general understanding of the world, choosing the right projection can significantly impact the accuracy and clarity of the information conveyed. By embracing the world of map projections, we gain a deeper appreciation for the challenges and intricacies of representing our planet on a flat surface.
![]()
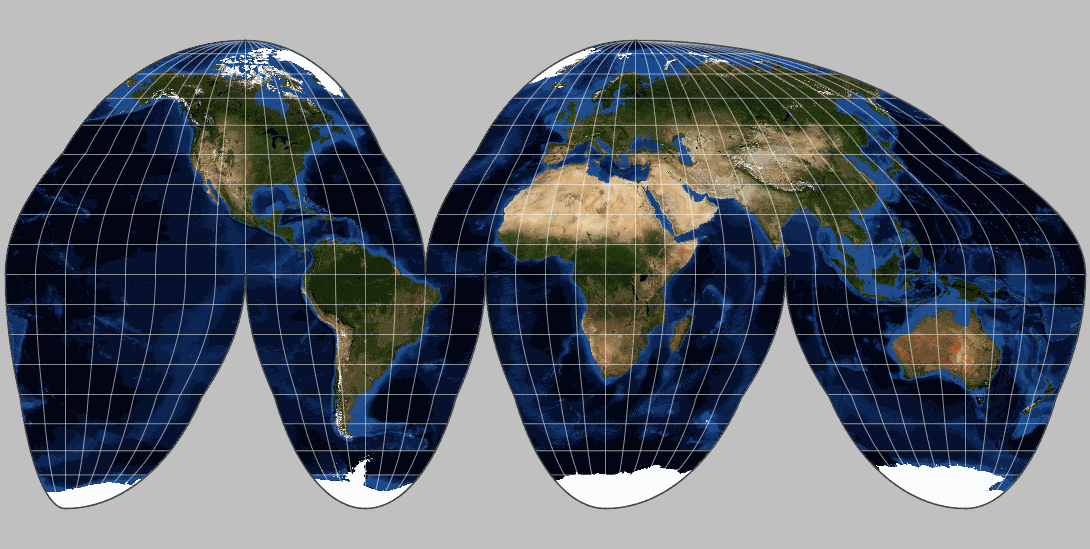


![50 Map Projections Types: A Visual Reference Guide [BIG LIST]](https://i.pinimg.com/736x/92/c0/c3/92c0c3a32f48481ca33ef54d5862f5a5.jpg)
Closure
Thus, we hope this article has provided valuable insights into Unveiling the World: A Comprehensive Guide to Map Projections. We hope you find this article informative and beneficial. See you in our next article!