Unveiling The Geometry Of Maps: Understanding Map Projections

Unveiling the Geometry of Maps: Understanding Map Projections

Related Articles: Unveiling the Geometry of Maps: Understanding Map Projections

Introduction

With great pleasure, we will explore the intriguing topic related to Unveiling the Geometry of Maps: Understanding Map Projections. Let’s weave interesting information and offer fresh perspectives to the readers.

Unveiling the Geometry of Maps: Understanding Map Projections

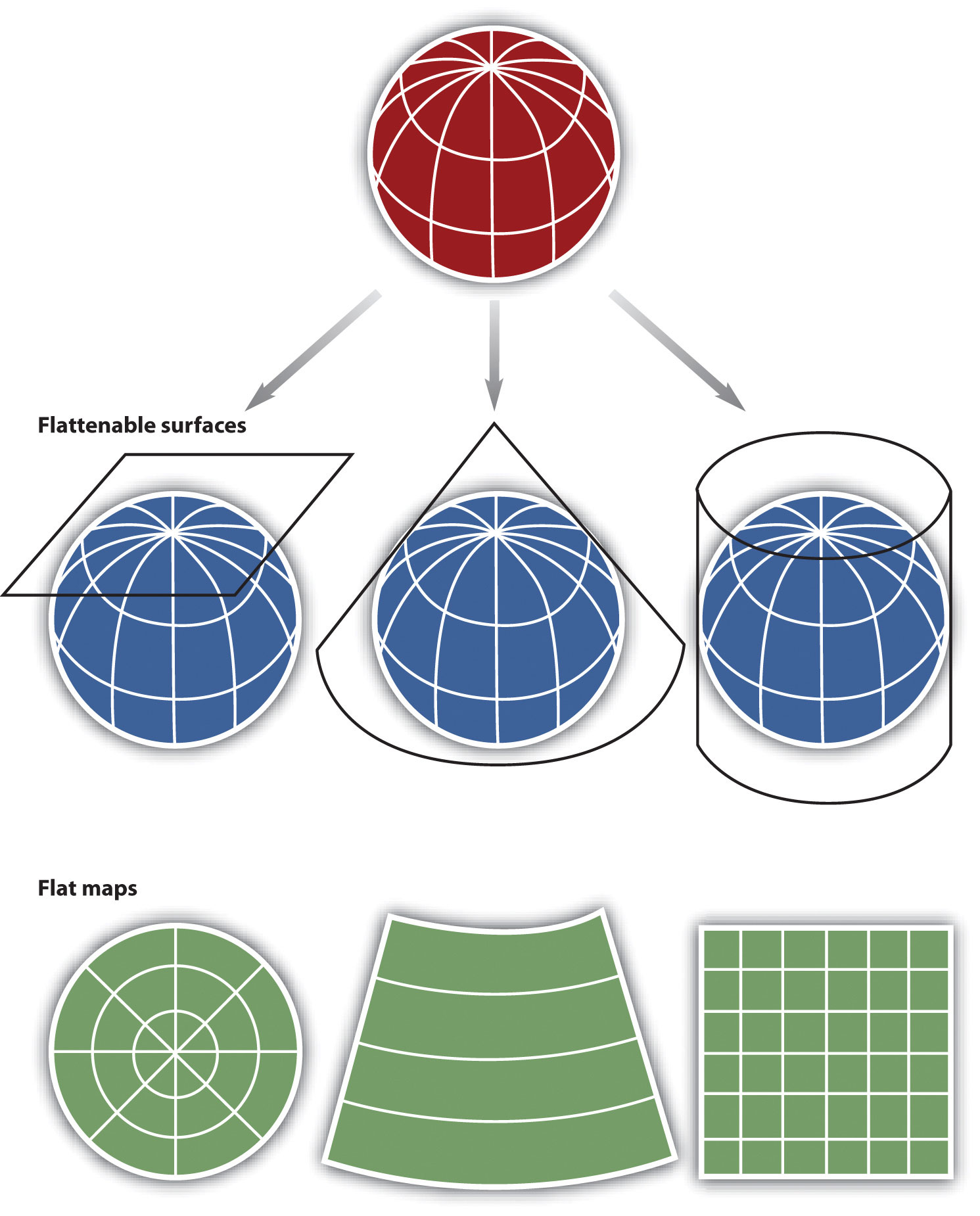
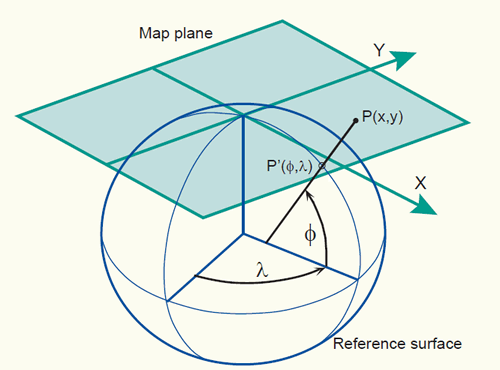
The Earth, a three-dimensional sphere, presents a challenge when attempting to represent it on a two-dimensional surface like a map. This is where map projections come into play. A map projection is a systematic transformation of the Earth’s curved surface onto a flat plane, enabling us to depict the globe in a comprehensible form. However, this transformation inevitably involves distortions, as it is impossible to faithfully represent all aspects of the Earth’s geometry on a flat surface.

Properties of Map Projections

The properties of a map projection define its characteristics and influence its suitability for specific applications. Understanding these properties is crucial for choosing the right projection for a particular purpose. Key properties include:

1. Preservation of Properties:

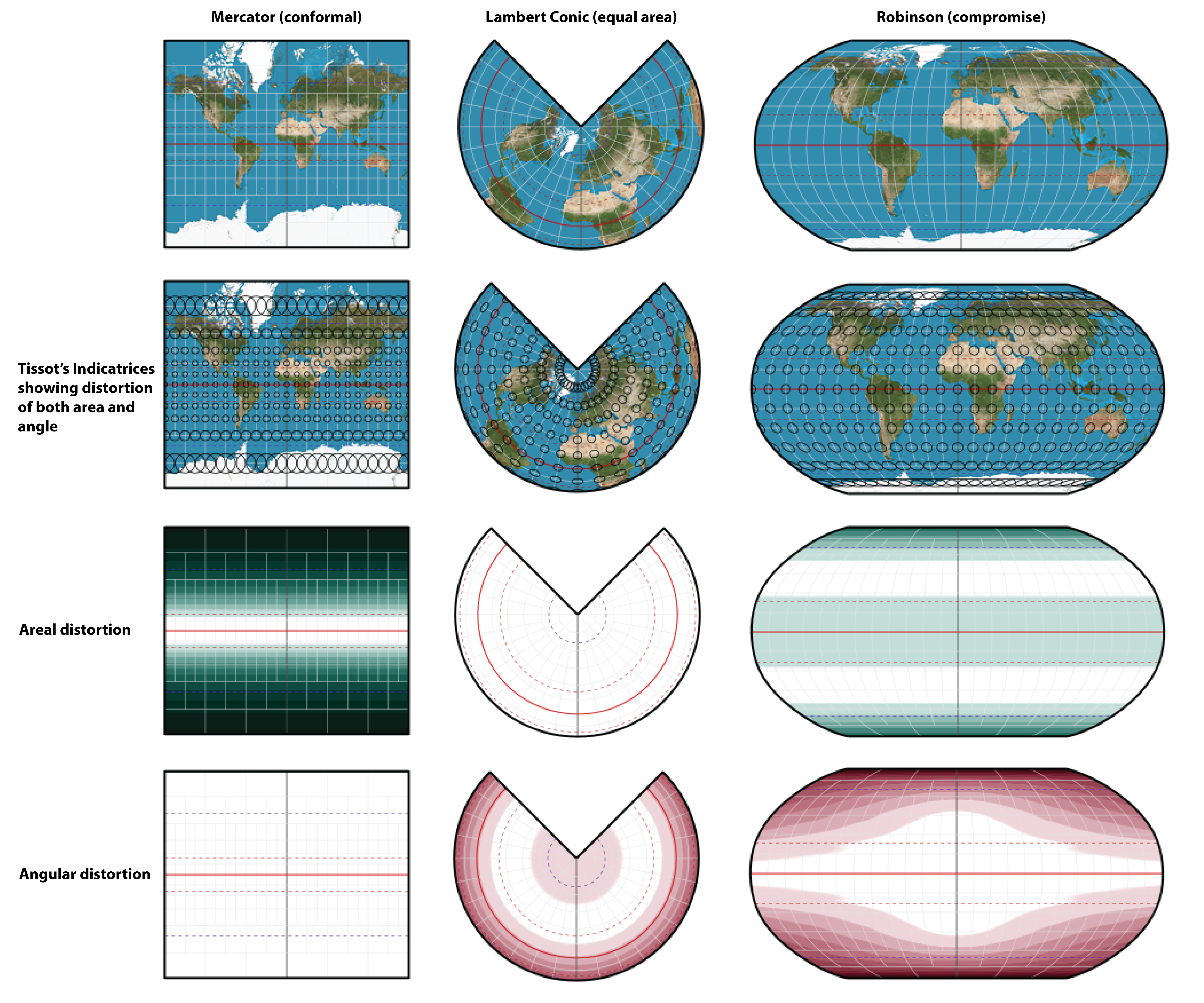
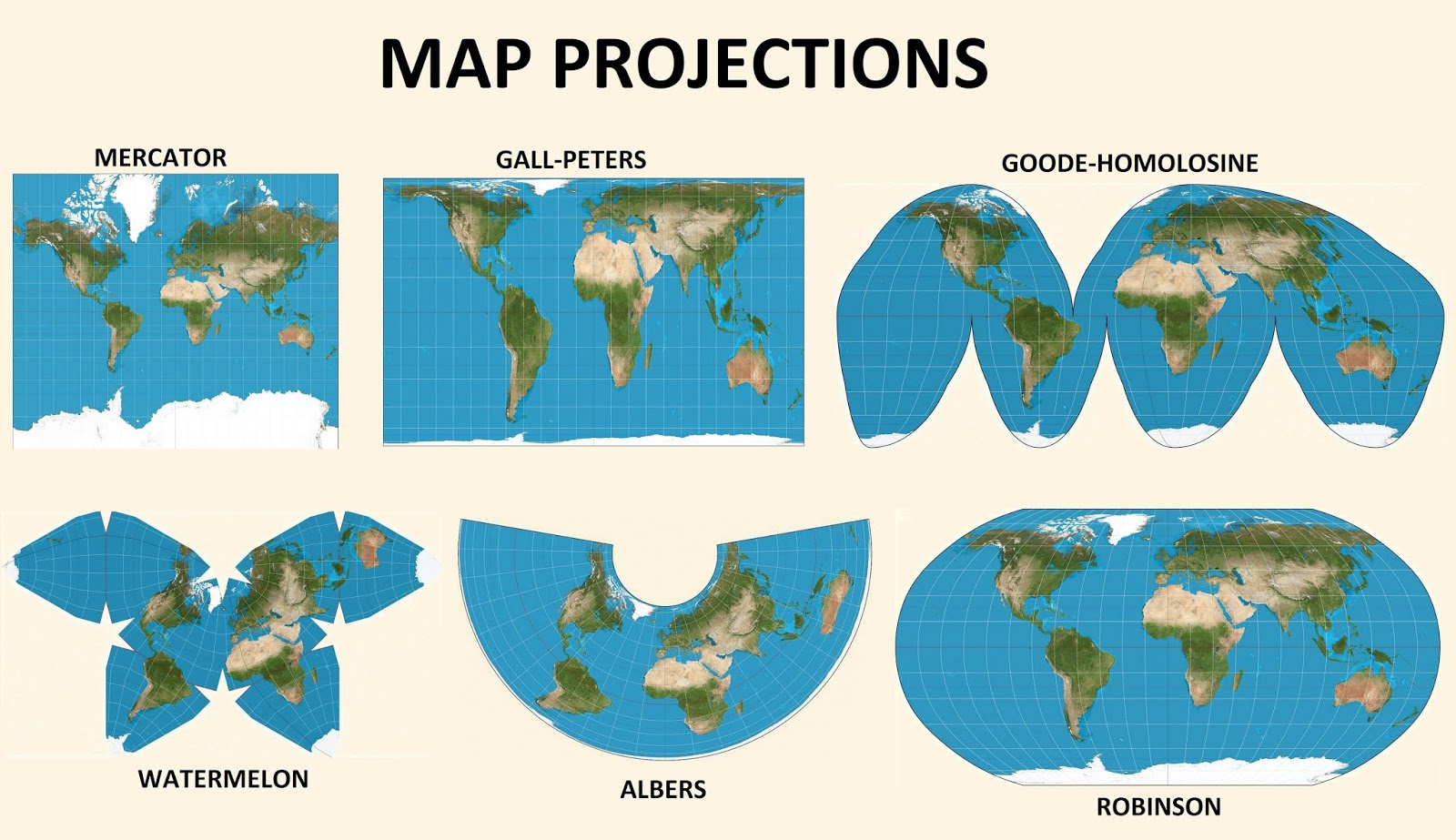
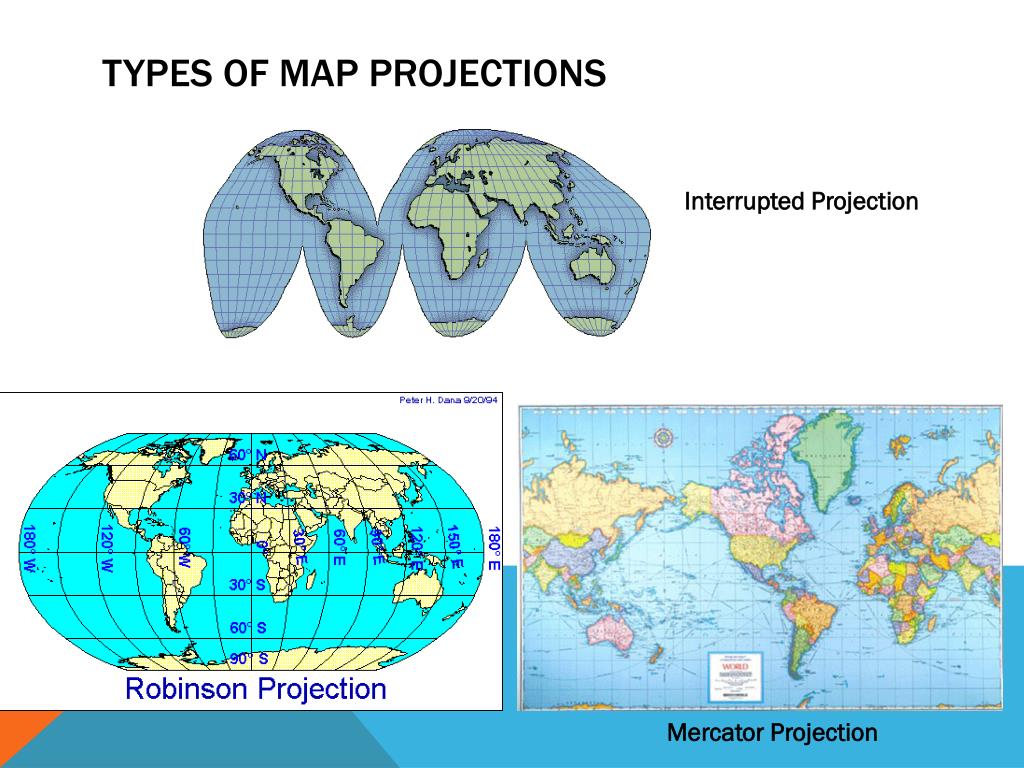
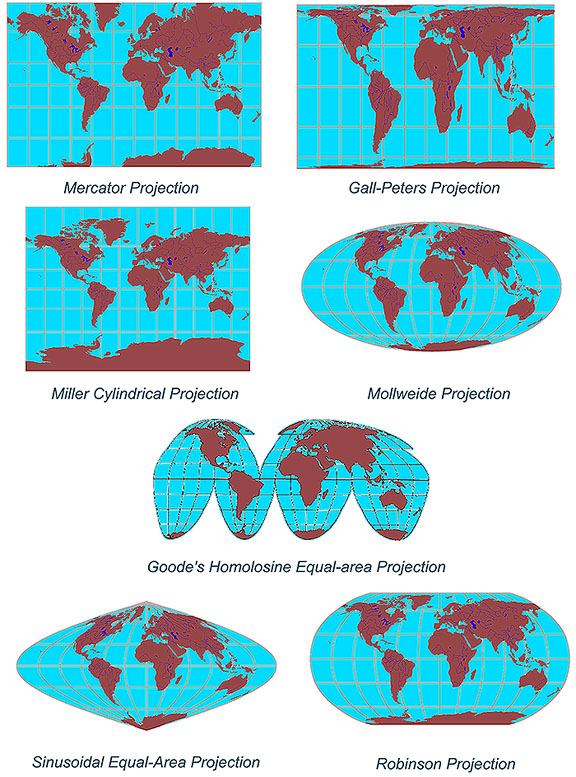
• Conformal Projection: These projections preserve angles, ensuring that shapes of small features are accurately represented. However, they distort areas, making larger features appear disproportionate. Examples include the Mercator projection, commonly used for navigation, and the Lambert Conformal Conic projection, often used for topographic maps.
• Equal-Area Projection: These projections preserve areas, meaning that the relative sizes of features on the map are accurate. However, they distort angles and shapes, making them unsuitable for applications requiring precise geometric representation. Examples include the Albers Equal-Area Conic projection, commonly used for thematic maps, and the Mollweide projection, often used for global maps.
• Equidistant Projection: These projections preserve distances from a specific point, usually a pole or the equator. While useful for representing distances from a central location, they distort both angles and areas. Examples include the Azimuthal Equidistant projection, used for navigation and mapping polar regions, and the Sinusoidal projection, often used for world maps.

2. Distortion Characteristics:

• Scale Distortion: This refers to the variation in the map scale across the projection. Scale distortion is inherent in all map projections, as the Earth’s curved surface cannot be perfectly flattened. Some projections, like the Mercator, exhibit significant scale distortion at higher latitudes, while others, like the Transverse Mercator, minimize distortion within a specific zone.
• Shape Distortion: This refers to the distortion of shapes, particularly of large features, on the map. Conformal projections minimize shape distortion, while equal-area projections introduce significant shape distortion.
• Area Distortion: This refers to the distortion of areas, affecting the relative sizes of features on the map. Equal-area projections preserve areas, while conformal projections introduce area distortion.

3. Projection Type:

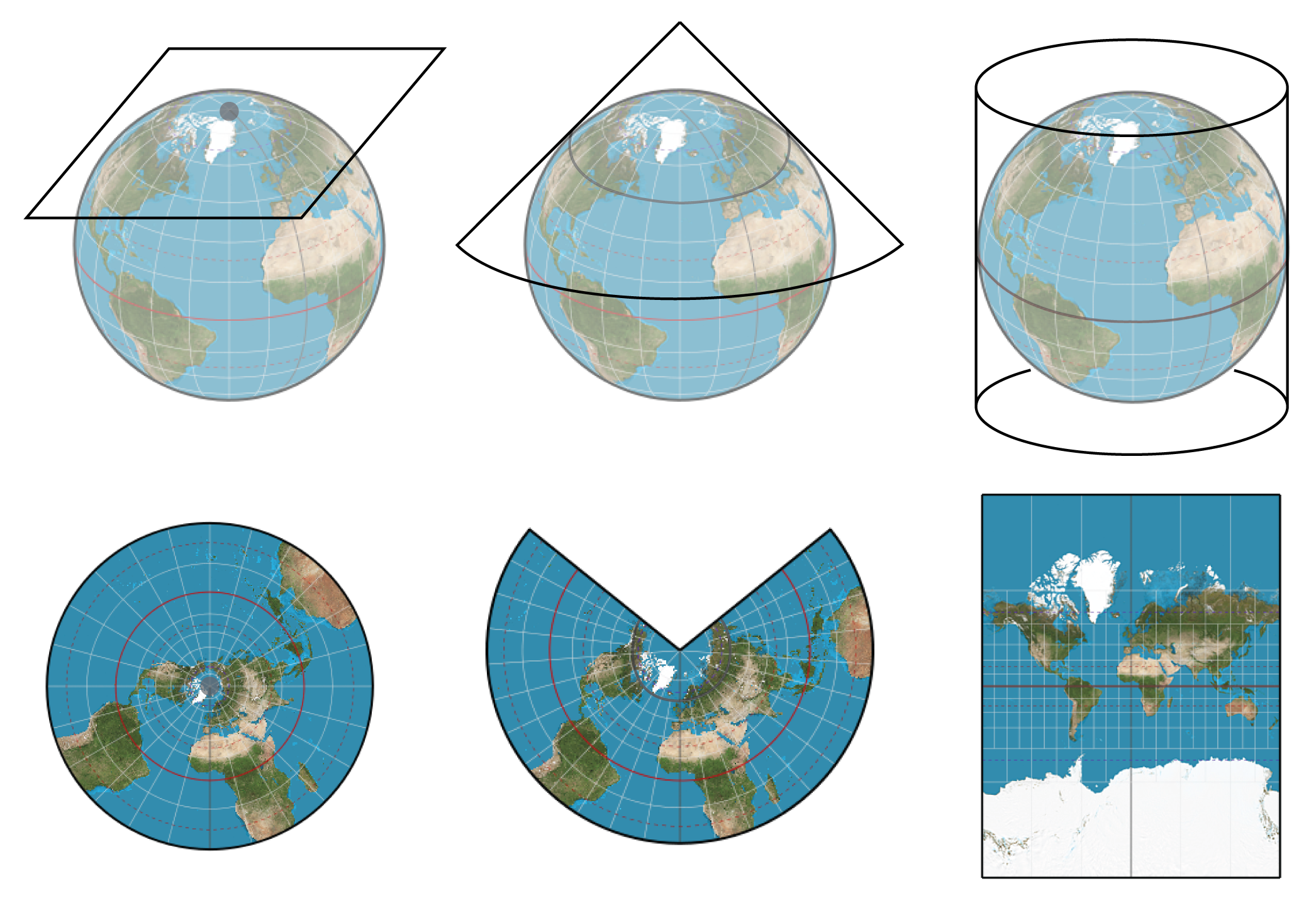
• Cylindrical Projection: These projections wrap a cylinder around the Earth, projecting features onto the cylinder’s surface. Examples include the Mercator and Transverse Mercator projections.
• Conic Projection: These projections project features onto a cone that intersects the Earth. Examples include the Lambert Conformal Conic and Albers Equal-Area Conic projections.
• Azimuthal Projection: These projections project features onto a plane tangent to the Earth. Examples include the Azimuthal Equidistant and Stereographic projections.

4. Standard Lines:

These lines on the map represent the lines of true scale, where distortion is minimized. For example, in a conic projection, the standard lines are the parallels along which the cone intersects the Earth.

Importance of Understanding Map Projections

Understanding the properties of map projections is crucial for various reasons:

• Accurate Interpretation: Choosing the right projection ensures that map information is interpreted correctly. A projection unsuitable for the task can lead to misinterpretations of distances, areas, shapes, and other geographical information.
• Effective Communication: Maps are used for communication and conveying spatial information. Selecting the appropriate projection enhances the clarity and accuracy of the message.
• Scientific Applications: In fields like geography, cartography, and remote sensing, map projections are fundamental tools for analyzing and visualizing spatial data.
• Navigation and Surveying: Accurate map projections are crucial for navigation, surveying, and other activities requiring precise spatial information.

FAQs about Map Projections

1. What is the most accurate map projection?

There is no single "most accurate" map projection. Accuracy depends on the specific application and the properties that need to be preserved. For example, a conformal projection is best for navigation, while an equal-area projection is best for representing areas accurately.

2. Why do map projections distort the Earth?

Map projections distort the Earth because it is impossible to perfectly flatten a curved surface onto a plane without introducing some form of distortion. The type and extent of distortion depend on the specific projection used.

3. How do I choose the right map projection?

The choice of projection depends on the intended use of the map. Consider the following factors:

• The geographic area being mapped
• The properties that need to be preserved (e.g., area, shape, distance)
• The intended application of the map

Tips for Understanding Map Projections

• Visualize the Projection: Try to visualize the relationship between the Earth’s curved surface and the projection surface (cylinder, cone, or plane).
• Examine Distortion Patterns: Pay attention to the distortion patterns in different projections, particularly near the poles and edges of the map.
• Compare Different Projections: Compare the characteristics of different projections to understand their strengths and weaknesses.
• Consult with Experts: If you are unsure about which projection to use, consult with a cartographer or other expert in spatial data.

Conclusion

Map projections are essential tools for representing the Earth’s curved surface on a flat plane. Understanding their properties is crucial for interpreting maps correctly, communicating spatial information effectively, and conducting scientific research. By carefully considering the specific application and the properties that need to be preserved, users can select the most appropriate projection for their needs, ensuring accurate and meaningful representation of geographical data.

Closure

Thus, we hope this article has provided valuable insights into Unveiling the Geometry of Maps: Understanding Map Projections. We appreciate your attention to our article. See you in our next article!