robinson projection map vs mercator
Related Articles: robinson projection map vs mercator
Introduction
With great pleasure, we will explore the intriguing topic related to robinson projection map vs mercator. Let’s weave interesting information and offer fresh perspectives to the readers.
Table of Content
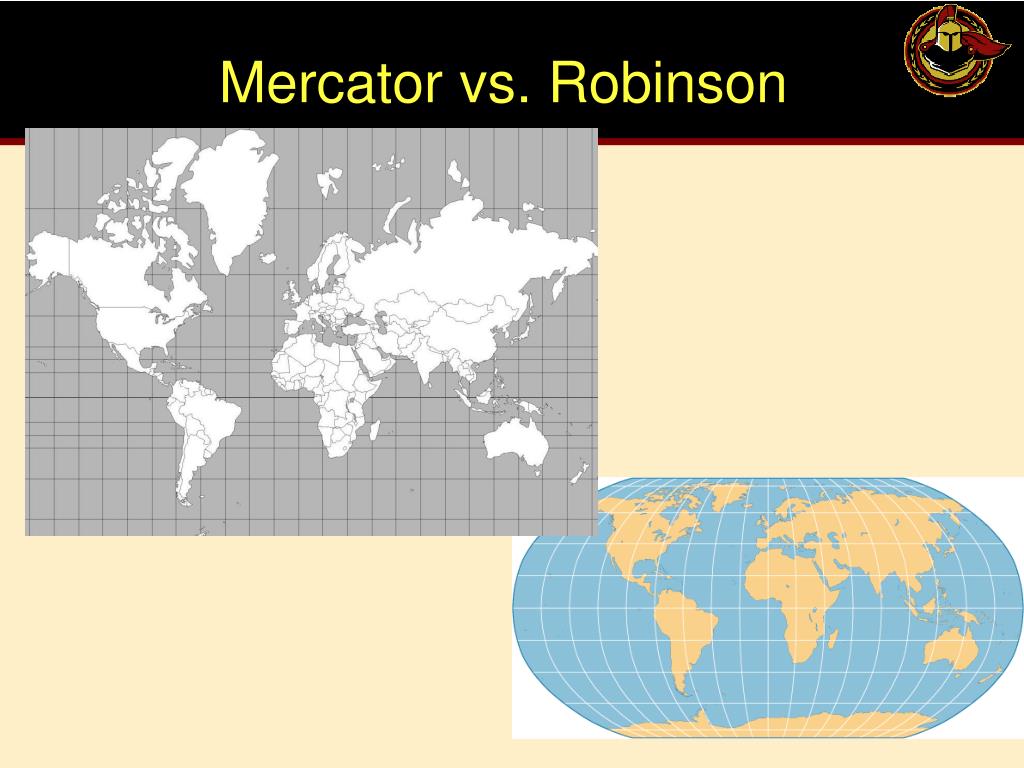
A Comparative Study: The Robinson Projection vs. the Mercator Projection
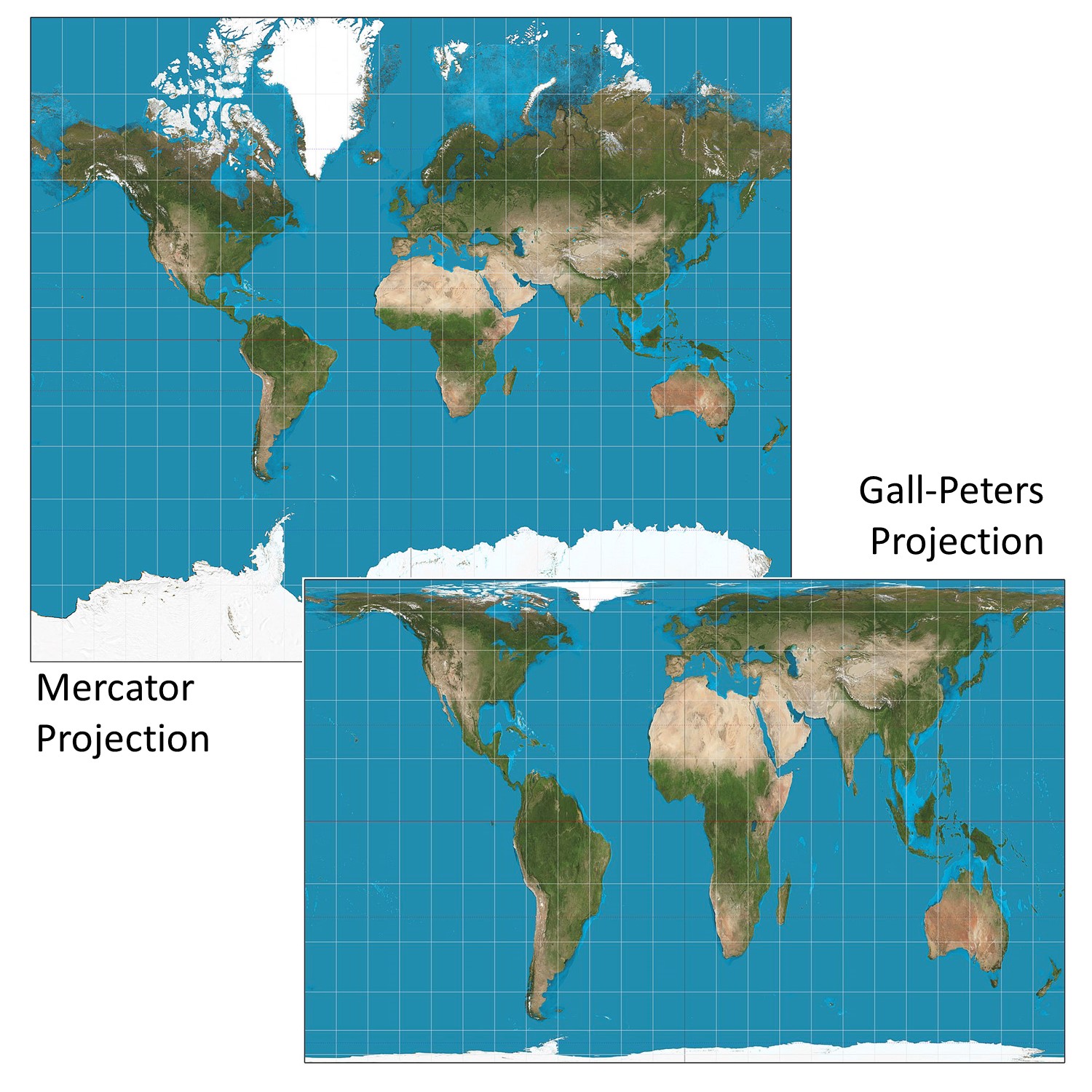
The world map is a ubiquitous tool, present in classrooms, offices, and homes. However, the seemingly simple act of representing our planet on a flat surface involves a fundamental challenge: accurately depicting the curved Earth on a two-dimensional plane. This necessitates the use of map projections, mathematical transformations that inevitably introduce distortions. Two prominent projections, the Robinson and the Mercator, have become widely recognized for their distinct characteristics and applications.
The Mercator Projection: A Legacy of Navigation
Developed by Flemish cartographer Gerardus Mercator in 1569, the Mercator projection has long been the standard for nautical charts. Its defining feature is the preservation of angles, ensuring that compass bearings remain accurate. This property makes the Mercator projection invaluable for navigation, as it allows sailors to plot straight lines representing constant compass headings.
However, the Mercator projection comes with a significant drawback: it distorts the size and shape of landmasses, particularly at higher latitudes. Greenland, for instance, appears larger than South America on a Mercator map, despite having a significantly smaller land area. This distortion is a consequence of the projection’s increasing scale as it moves away from the equator, leading to a disproportionate exaggeration of polar regions.
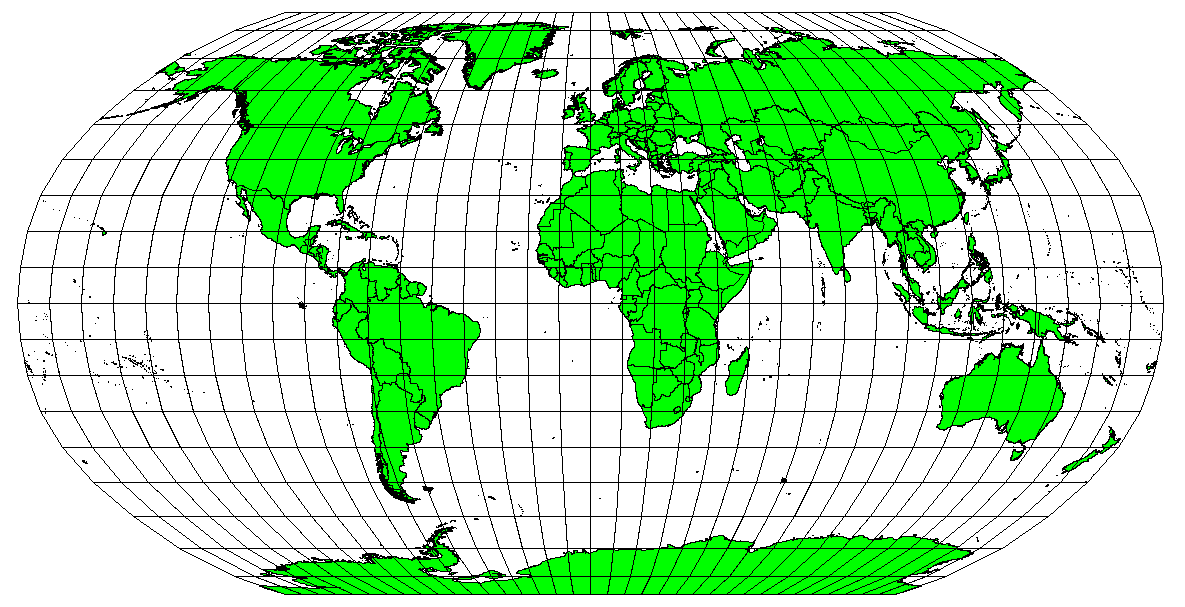
The Robinson Projection: A Compromise for Balance
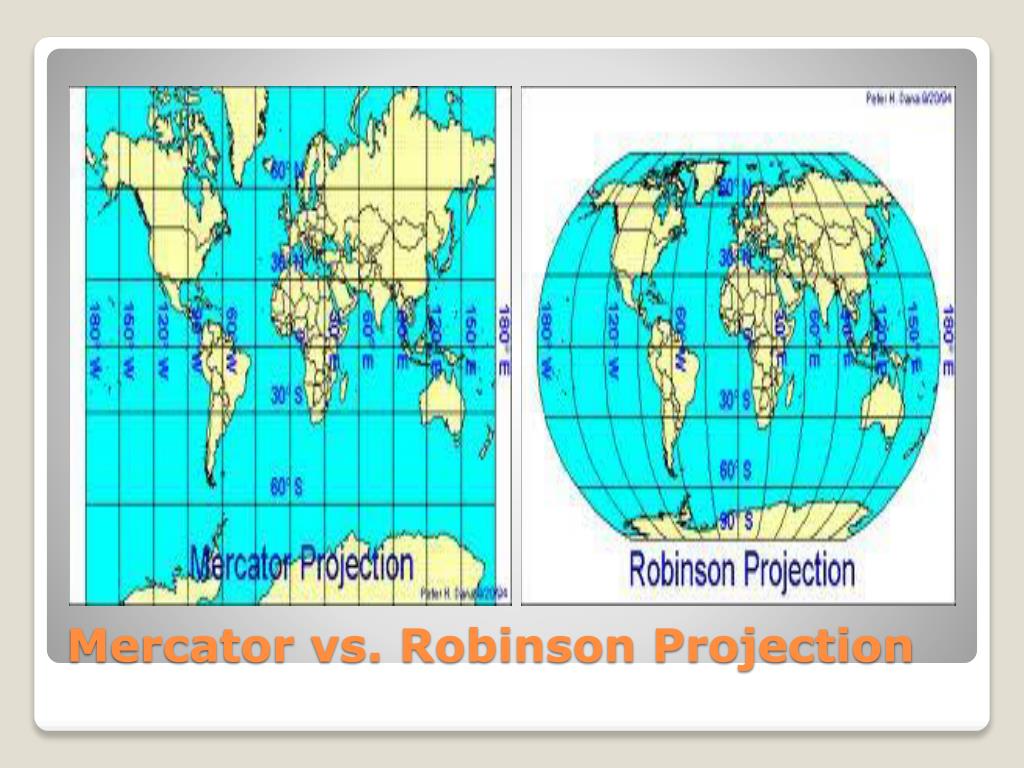
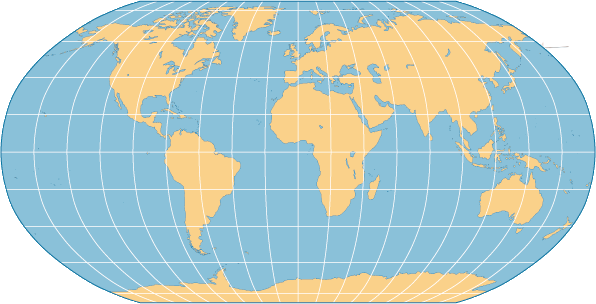
Introduced in 1963 by American cartographer Arthur H. Robinson, the Robinson projection aims to strike a balance between accuracy and visual appeal. Unlike the Mercator, the Robinson projection does not preserve angles or maintain a constant scale. Instead, it utilizes a compromise approach, minimizing distortions in areas like the equator and the poles.
The Robinson projection achieves this balance by distorting both area and shape, but to a lesser degree than the Mercator. This results in a map that is visually pleasing and provides a more realistic representation of the relative sizes of continents. However, it also means that the Robinson projection is not ideal for navigation, as compass bearings are not accurately represented.
A Comparative Analysis: Strengths and Weaknesses
The choice between the Robinson and Mercator projections ultimately depends on the intended purpose of the map. For navigation, the Mercator projection remains the preferred choice due to its preservation of angles. However, its significant distortion of landmasses makes it unsuitable for applications that require accurate representations of size and shape.
The Robinson projection, on the other hand, offers a more balanced representation of the world, minimizing distortions in both area and shape. This makes it suitable for general-purpose maps, atlases, and educational purposes. However, its compromise approach means that it does not excel in any specific area, lacking the navigational accuracy of the Mercator or the precise area representation of other projections.
Beyond the Two: A Broader Perspective
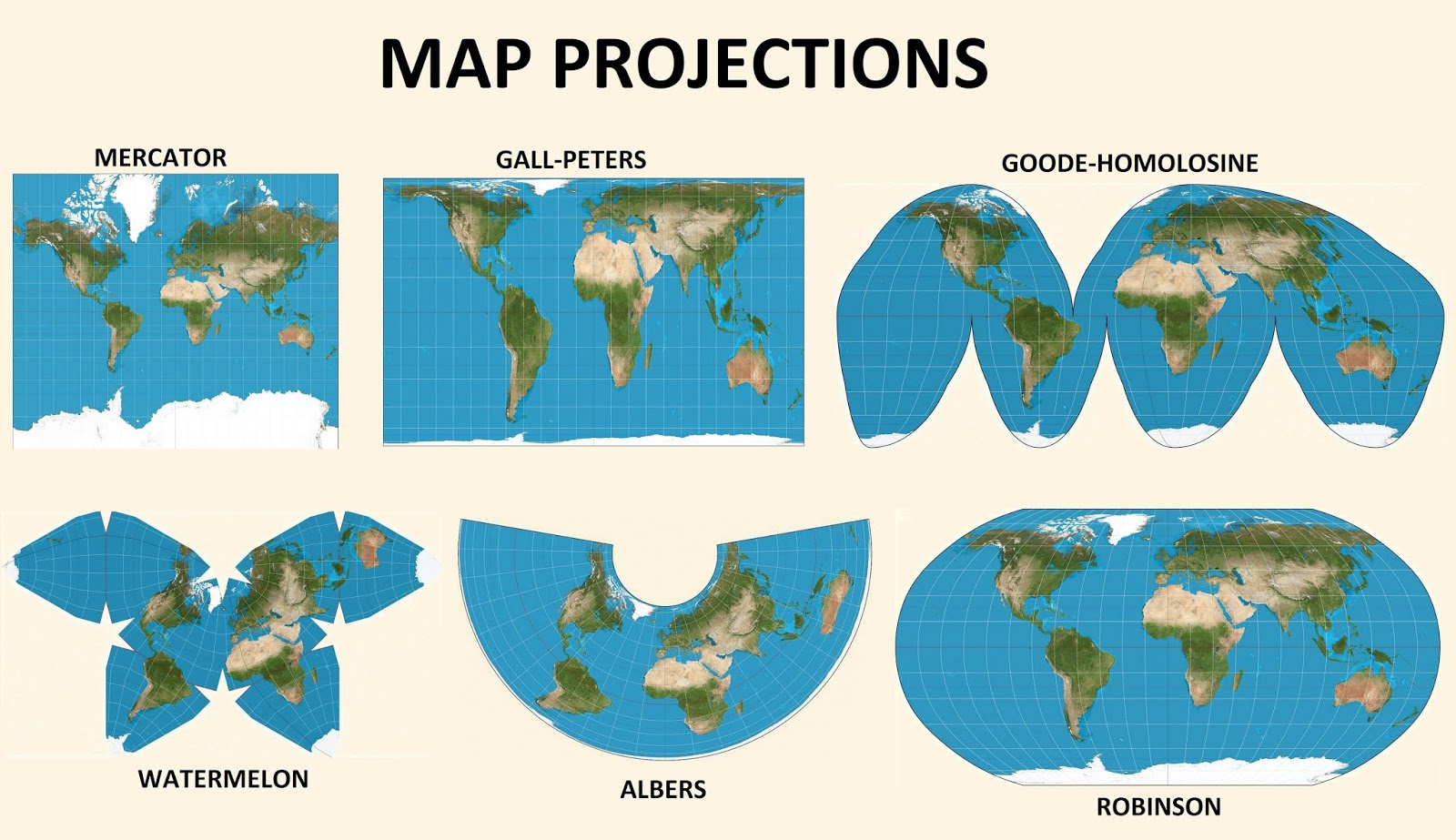
While the Robinson and Mercator projections are widely recognized, a multitude of other map projections exist, each with its own strengths and weaknesses. Some projections, like the Winkel Tripel, prioritize accurate area representation, while others, like the Mollweide, emphasize the preservation of shapes. The choice of projection ultimately depends on the specific requirements of the map and its intended use.
FAQs: Addressing Common Questions
Q: Which projection is more accurate, the Robinson or the Mercator?
A: Neither projection is inherently more accurate. Both introduce distortions, but in different ways. The Mercator preserves angles, making it accurate for navigation, while the Robinson minimizes distortions in area and shape, providing a more balanced representation of the world.
Q: Why is the Mercator projection still widely used despite its distortions?
A: The Mercator projection has a long history and is deeply ingrained in maritime navigation. Its preservation of angles makes it invaluable for sailors, even though it distorts the size and shape of landmasses.
Q: What are some alternatives to the Robinson and Mercator projections?
A: Numerous other projections exist, each with its own strengths and weaknesses. Some notable alternatives include the Winkel Tripel, Mollweide, and Goode Homolosine projections.
Tips for Choosing the Right Projection:
- Consider the purpose of the map: If navigation is the primary goal, the Mercator projection is suitable. For general-purpose maps, the Robinson or other projections that minimize distortions are better choices.
- Understand the limitations of each projection: Every projection introduces some level of distortion. Choose a projection that minimizes distortions in the areas most relevant to your map.
- Explore different projections: Familiarize yourself with a variety of projections to understand their unique characteristics and choose the one that best suits your needs.
Conclusion: The Ongoing Evolution of Cartography
The Robinson and Mercator projections represent two significant milestones in the history of cartography. While the Mercator projection continues to play a vital role in navigation, the Robinson projection offers a more balanced representation of the world, making it suitable for a wider range of applications. As cartographic techniques continue to evolve, new projections are being developed, further refining our ability to represent the Earth on a flat surface. This ongoing evolution ensures that maps remain a powerful tool for understanding and exploring our planet.



Closure
Thus, we hope this article has provided valuable insights into robinson projection map vs mercator. We appreciate your attention to our article. See you in our next article!